
 |
Integrals of the Logarithm, log(x) or ln(x), with polynomials and rational functions |
The attached .pdf file is a lengthy study of the integration of functions involving combinations of logarithms and polynomials, such as log(x+1)/(x^2+1).
I came across one such problem in the 2005 MMA William Putnam competition, managed to solve it, and generalised. The techniques use complex contour integration and summation of infinite series. Catalan's constant features in several integrals.
In the paper I review many of the published definite and indefinite integrals of this type, and derive several which are not in the extensive classic collection of Gradshteyn and Ryzhik. Most examples are worked out in full, and so may be useful to students learning the techniques of complex integration.
In many cases it turns out that, by integrating functions of the form P(x) ln(x), the definite integral of P(x) can be obtained, where P(x) is a polynomial or rational function.
Download whole document (pdf file).

Here are a few sample results. First the solution to the above 2005 Putnam competition question:
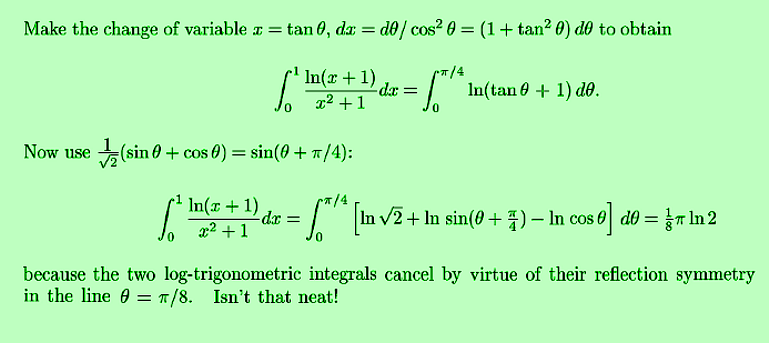
In my article I generalise from this special case. Most general cases cannot be integrated in closed form merely by appeal to symmetry, so complex contour integration is required. There is a notable relation between the definite integrals (0 to 1) and (0 to infinity) given as follows, where G is Catalan's constant:

Fortunately many of the instance for p a positive integer can be evaluated in closed form, as this table below shows. However, I do not know whether the integrals with fractional powers can be evaluated in closed form.

Below is a selection of results from the article:


and my most hard-won result is

For many more log-rational integrals, plus details of their computation, download the full text (pdf file).
Recently I came across another integral involving ln(x^2+1). It is multiplied by a Bessel function of order 1, and the value of the integral is a modified Bessel function:

I lifted this intriguing result direct from a 1994 paper by Lucas and Stone of Harvard -- no credit to me at all!
